How did he approach the problem in the first place, and how did he eliminate 5?
-
Module 0 Week 3 Day 12 Your Turn Explanation Part 2
Although I understand the last bit of the solution to the problem, but I didn't understand how he apporached the problem in the first place. And how did he eliminated 5 from 3 * 2 * 5 = 30.
[Can you explain me the problem from the start please?]
Thank you for your help. -
Hi @fabulousgrizzly!
That's totally okay if you didn't get everything! We can explain it to you now

To begin with, I would like to discuss the following diagram:
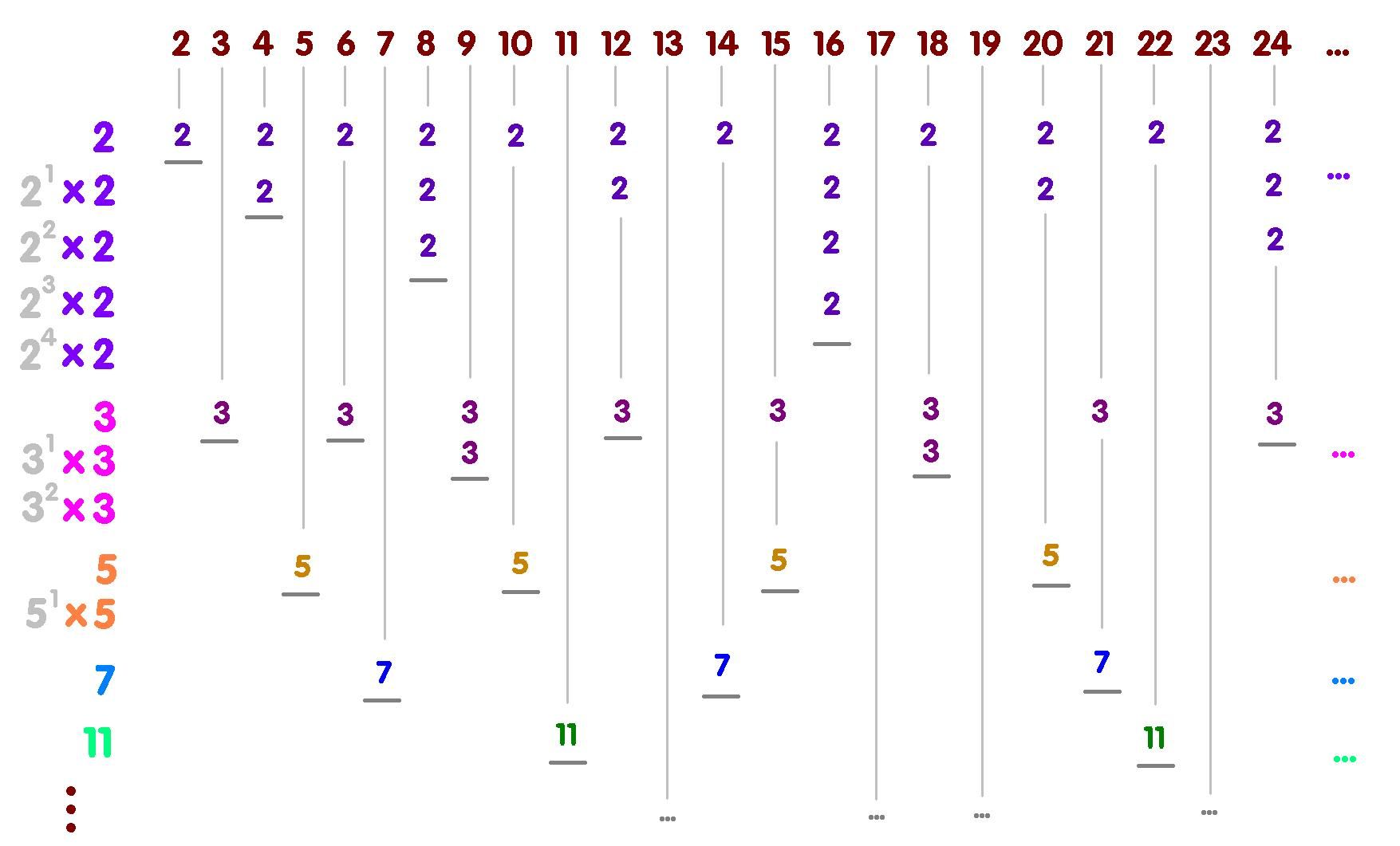
In the diagram, under each number, I've listed all of that number's prime factors along with the quantity that equals the power of this prime factor in the number \((\)e.g. under \(12\) there are \(\text{\color{darkred}two } 2s\) and \(\text{\color{darkblue}one } 3,\) since \(12=2^{\color{darkred}\bf2}\times 3^{\color{blue}\bf 1}).\)As you can see, while the number \(\bf 21\) gets one factor \(\color{green}\bf 7\) in addition to \(3,\) the number \(\bf 12\) already has two \({\bf \color{green}2}s\) with the same \(3.\) When we look at two numbers like \({\bf 21}=3^1\times {\color{green}\bf 7^1}\) and \({\bf 12}=3^1\times {\color{green}\bf 2^2},\) the last one will have more factors than the first one, since the power of the prime numbers in the different parts are different. Or, put in a more understandable way, \(\bf 21\) has \((1+1)\times ({\color{green}\bf 1}+1)=4\) factors, while \(\bf 12\) has \((1+1)\times ({\color{green}\bf 2}+1)=6\) factors.
To reiterate, if we want more factors, we should put as many (and more small) prime factors as we can in the number before our limit: \(30.\) For example, in the number \(\bf 21,\) we can change \(\color{green}\bf 7\) to two \({\bf \color{green}2}s\) and get the number \(\bf 12\) which has more factors.So now, every time when we find big prime factors in the numbers, we will try to change them to a larger amount of smaller ones. There are a few cases: when the number has less than 3 prime factors (one or two), we can use \(2s\) and \(3s\) to replace the bigger ones and get more factors at the end. There is also the case where we try to put three different prime numbers into the number: \(30=2\times 3\times 5.\) However, we are not allowed to use any number that greater or equal to 30. So we are allowed to use only numbers that have one or two prime factors; that's why Prof. Loh said that we can forget about \(5,\) because we will be able to use only \(2s\) and \(3s\) to get more factors.
Now, the only thing left is to count the number with the prime factors \(2\) and \(3\) that has the biggest number of factors.
I guess that this is the part you said you understood.
If I am wrong, don't hesitate to ask me about it! I am always open for questions!
Happy Learning!
-
I think you just copy and paste -_-