Voice typo?

[The Blade Dancer]

At timestamp 1:10 it seemed like Prof. Loh just repeated what he said. Also when or where can I find proof of why inscribed angles= 1/2 the angles they inscribe? I forgot sorry.

[nastya]

@The-Darkin-Blade Hi there!
Thank you for mentioning about this voice typo! We will definitely take a look and think about what to do about it!
Now, let me answer your question about inscribed angles.
The formal wording of this theorem goes like this:
 
Inscribed Angle Theorem:
Any angle that is inscribed in a circle is half of the central angle that subtends the same arc on the circle.
 
Proof:
Let our inscribed angle be $\angle BAC$ and the central angle $\angle BOC.$
There are two cases for the position of the inscribed angle relative to the central angle.
 
Case 1:

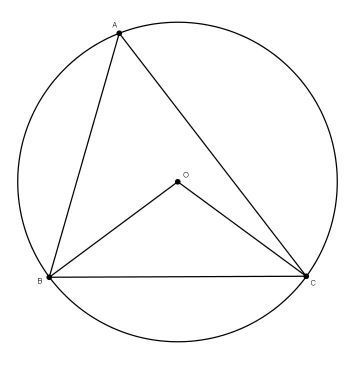
Let's draw one more radius, $\overline{OA}.$ Now we have three isosceles triangles: $\bigtriangleup AOB,$ $\bigtriangleup AOC$ and $\bigtriangleup BOC.$ So the the angles at the base are equal. Let $\alpha = \angle BAO=\angle ABO,$ $\beta = \angle CAO=\angle ACO$ and $\gamma= \angle BCO=\angle CBO.$ So the $\angle BAC = \alpha+\beta.$

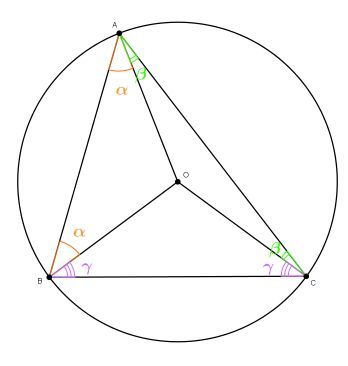
Let's now find the measure of the angle $\angle BOC:$
The sum of the angles in any triangle is equal to $180^{\circ},$ so $\angle BOC=180^{\circ}-2\gamma$ and also $\angle BAC+\angle ABC+\angle ACB=180^{\circ}.$
On the other hand, $\text{sum of the angles in }\triangle ABC=2\alpha+2\beta+2\gamma=180^{\circ},$ hence $2\alpha+2\beta = 180^{\circ}-2\gamma=\angle BOC.$ And we got what we wanted: $\angle BOC=2\alpha+2\beta=2(\alpha+\beta)=2\angle BAC.$
So we are done!
 
Case 2:
Now, if you want, you can try to prove this case yourself, using the ideas from the proof of the first case

Let's draw one more radius, $\overline{OA}.$ Now we have three isosceles triangles: $\bigtriangleup AOB,$ $\bigtriangleup AOC$ and $\bigtriangleup BOC.$ So the angles at the base are equal. Let $\alpha = \angle BAO=\angle ABO,$ $\beta = \angle CAO=\angle ACO$ and $\gamma= \angle BCO=\angle CBO.$ So the $\angle BAC = \alpha-\beta.$

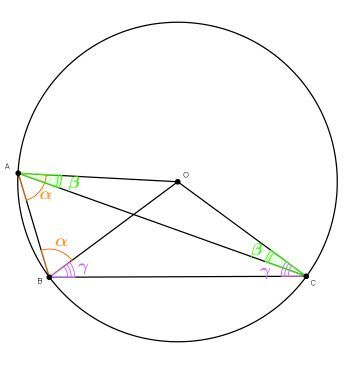
Let's now find the measure of the angle $\angle BOC:$
The sum of the angles in any triangle is equal to $180^{\circ},$ so $\angle BOC=180^{\circ}-2\gamma$ and also $\angle BAC+\angle ABC+\angle ACB=180^{\circ}.$
On the other hand, $\text{sum of the angles in }\triangle ABC=(\alpha-\beta)+(\alpha+\gamma)+(\gamma-\beta) = 2\alpha-2\beta+2\gamma=180^{\circ},$ hence $2\alpha-2\beta = 180^{\circ}-2\gamma=\angle BOC.$ And we got what we wanted: $\angle BOC=2\alpha-2\beta=2(\alpha-\beta)=2\angle BAC.$
So we are done again, using the same algorithm!

[debbie]

@nastya $\bigtriangleup ACO$